CHAPTER 2
CONCURRENCY
2.1 Perpendicular Bisectors
This chapter is concerned with concurrent lines associated with a triangle. A family of lines is concurrent at a point P if all members of the family pass through P.
In preparation, we need a few additional facts about parallel lines.
Theorem 2.1.1. Let l1 and l2 be parallel lines, and suppose that m1 and m2 are lines with m1 ⊥ l1 and m2 ⊥ l2. Then m1 and m2 are parallel.
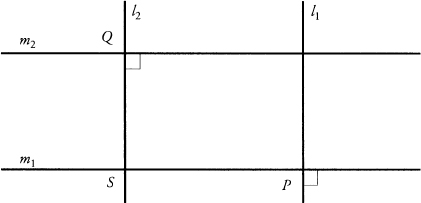
Proof. Let P be the point l1 ∩ m1 and let Q be the point l2 ∩ m2. By the parallel postulate, l1 is the only line through P parallel to l2, and so m1 is not parallel to l2 and consequently must meet l2 at some point S. In other words, m1 is a transversal for the parallel lines l1 and l2. Since the sum of the adjacent interior angles is 180°, it follows that l2 must be perpendicular to m1.
But l2 is also perpendicular to m2 and so m1 and m2 must be parallel.
In the above proof, if we interchange l1 and m1 and l2 and m2, we have:
Corollary 2.1.2. Suppose that l1 ⊥ m1 and l2 ⊥ m2. Then l1 and l2 are parallel if and only if m1 and m2 are parallel.
One of the consequences of this corollary is that if two line segments intersect, then their perpendicular bisectors must also intersect. This fact is crucial in the following theorem, the proof of which also uses the fact that the right bisector of a segment can be characterized as being the set of all points that are equidistant ...
Get Classical Geometry: Euclidean, Transformational, Inversive, and Projective now with the O’Reilly learning platform.
O’Reilly members experience books, live events, courses curated by job role, and more from O’Reilly and nearly 200 top publishers.

