Analysis of Complex Networks

14.5 Classification of Elementary ({2, 3}, 5)-Polycycles

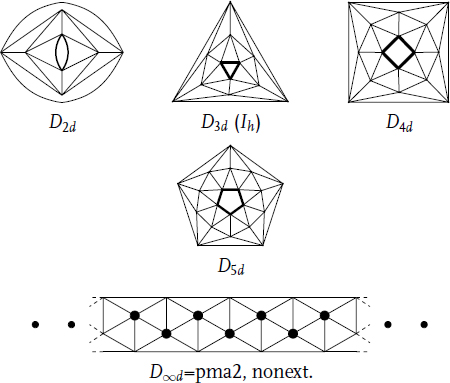
For 2 ≤ m ≤ ∞, call snub m-antiprism the ({3},5)_simp-polycycle with two m-gonal holes separated by 4m 3-gonal proper faces. This polycycle is nonextensible if and only if m ≤ 4. Its symmetry group, D_md, coincides with the symmetry group of an underlying 5-valent plane graph if and only if m =3. See below for pictures of a snub m-antiprism for m = 2, 3, 4, 5, and ∞ (see [4], p. 119 for a formal definition):

Theorem 14.5 The list of elementary ({2, 3},5)-polycycles consists of:

(i) 57 sporadic ({2, 3},5)_simp-polycycles, given in Appendix 2.

(ii) The following 3 infinite ({2, 3},5)_simp-polycycles:

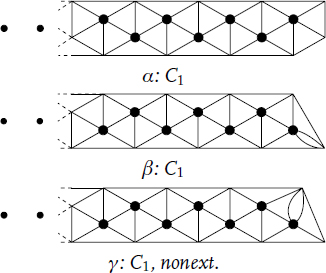
(iii) 6 infinite series of ({2, 3},5)_simp-polycycles with one hole (obtained by concatenating endings of a pair of polycycles, given in (ii); see Figure 14.2 for the first 4 polycycles).

(iv) The infinite series of snub m-antiprisms for 2 ≤ m ≤ ∞ and its nonorientable quotient for m odd.

Proof. Take an elementary ({2, 3},5)_simp-polycycle P that, by Proposition 14.2, is kernel-elementary. If its kernel is empty, then P is simply an r-gon with r ∈{2, 3}. If the kernel is reduced to a vertex, then P is simply a 5-tuple of 2-, 3-gons. If three edges e_i = {v_i–1, v_i}, i =1,..., 3 are part of the kernel with e₁, e₂ and e₂, e₃ not part of an ...